Math 411: Honours Complex Variables - University of Alberta

z0 ∈ D, the germ of f at z0—denoted by 〈f〉z0—is the collection of all function
elements (E,g) such that z0 ∈ E and there is a neighbourhood U ⊂ D∩E of z0 such
that f(z) = g(z) for all z ∈ U.
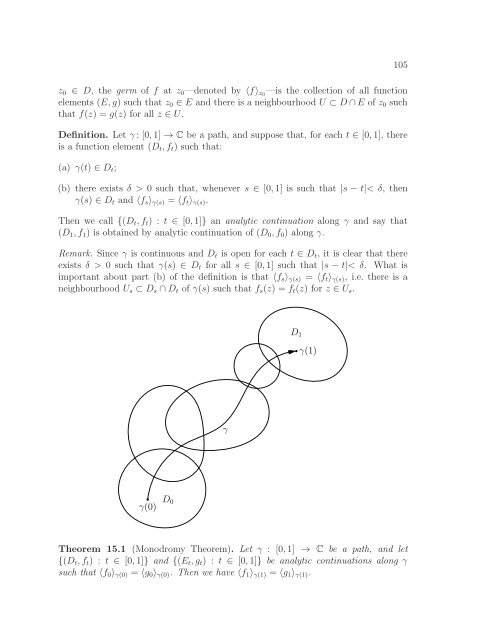
Definition. Let γ: [0,1] → C be a path, and suppose that, for each t ∈ [0,1], there
is a function element (Dt,ft) such that:
(a) γ(t) ∈ Dt;
(b) there exists δ > 0 such that, whenever s ∈ [0,1] is such that |s − t|< δ, then
γ(s) ∈ Dt and 〈fs〉γ(s) = 〈ft〉γ(s).
Then we call {(Dt,ft) : t ∈ [0,1]} an analytic continuation along γ and say that
(D1,f1) is obtained by analytic continuation of (D0,f0) along γ.
Remark. Since γ is continuous and Dt is open for each t ∈ Dt, it is clear that there
exists δ > 0 such that γ(s) ∈ Dt for all s ∈ [0,1] such that |s − t|< δ. What is
important about part (b) of the definition is that 〈fs〉γ(s) = 〈ft〉γ(s), i.e. there is a
neighbourhood Us ⊂ Ds ∩Dt of γ(s) such that fs(z) = ft(z) for z ∈ Us.
γ(0)
D0
γ
Theorem 15.1 (Monodromy Theorem). Let γ : [0,1] → C be a path, and let
{(Dt,ft) : t ∈ [0,1]} and {(Et,gt) : t ∈ [0,1]} be analytic continuations along γ
such that 〈f0〉γ(0) = 〈g0〉γ(0). Then we have 〈f1〉γ(1) = 〈g1〉γ(1).
D1
γ(1)
105