Chapter 5: Motion along a line
Chapter 5: Motion along a line
Chapter 5: Motion along a line
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
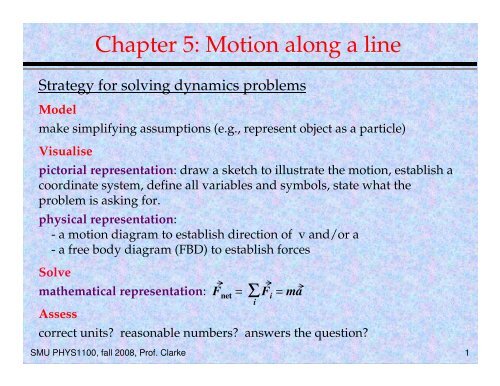
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Strategy for solving dynamics problems<br />
Model<br />
make simplifying assumptions (e.g., represent object as a particle)<br />
Visualise<br />
pictorial representation: draw a sketch to illustrate the motion, establish a<br />
coordinate system, define all variables and symbols, state what the<br />
problem is asking for.<br />
physical representation:<br />
- a motion diagram to establish direction of v and/or a<br />
- a free body diagram (FBD) to establish forces<br />
Solve<br />
mathematical representation: F net = F i = ma<br />
Assess<br />
correct units? reasonable numbers? answers the question?<br />
Σ i<br />
SMU PHYS1100, fall 2008, Prof. Clarke 1
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Newton’s 2 nd law must be treated as a vector equation!<br />
F Σ net = Fi = ma<br />
i<br />
is really 3 equations, one for each component.<br />
F Σ net,x = Fi,x = max i<br />
F Σ net,y = Fi,y = may i<br />
F Σ net,z = Fi,z = maz i<br />
and we must solve each separately.<br />
In this chapter, problems are in 2-D; z-components are zero.<br />
SMU PHYS1100, fall 2008, Prof. Clarke 2
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Definition: An object is in equilibrium when F net = 0, and thus<br />
when a = 0.<br />
If v = 0 too, equilibrium is static.<br />
example: If A exerts a force of 100 N west and B exerts a force<br />
of 120 N south, what force must C exert to maintain the knot<br />
in static equilibrium?<br />
A<br />
B<br />
C<br />
SMU PHYS1100, fall 2008, Prof. Clarke 3
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Newton’s 2 nd Law:<br />
broken up into components:<br />
F Σ net = Fi = T1 + T2 + T3 = ma = 0<br />
i<br />
x: F Σ net,x = Fi,x = −T1 + T3 cosθθθθ = 0<br />
i<br />
� T 3 cosθθθθ = T 1<br />
y: F Σ net,y = Fi,y = −T2 + T3 sinθθθθ = 0<br />
i<br />
� T 3 sinθθθθ = T 2<br />
divide (2) by (1) to get:<br />
tanθθθθ = T 2 /T 1<br />
SMU PHYS1100, fall 2008, Prof. Clarke 4<br />
(1)<br />
(2)<br />
(1) 2 + (2) 2 �<br />
T3 cos2θθθθ + T3 sin2θθθθ = T3 (cos2θθθθ + sin2 2 2<br />
2<br />
θ θ θ θ )<br />
2 2 2<br />
= T3 = T1 + T2 T 1<br />
y<br />
T 2<br />
θθθθ<br />
T 3<br />
T 3 cosθθθθ<br />
T 3 sinθθθθ<br />
x
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Newton’s 2 nd Law:<br />
broken up into components:<br />
F Σ net = Fi = T1 + T2 + T3 = ma = 0<br />
i<br />
x: F Σ net,x = Fi,x = −T1 + T3 cosθθθθ = 0<br />
i<br />
� T 3 cosθθθθ = T 1<br />
y: F Σ net,y = Fi,y = −T2 + T3 sinθθθθ = 0<br />
i<br />
� T 3 sinθθθθ = T 2<br />
divide (2) by (1) to get:<br />
tanθθθθ = T 2 /T 1<br />
SMU PHYS1100, fall 2008, Prof. Clarke 5<br />
(1)<br />
(2)<br />
(1) 2 + (2) 2 �<br />
T3 cos2θθθθ + T3 sin2θθθθ = T3 (cos2θθθθ + sin2 2 2<br />
2<br />
θ θ θ θ )<br />
2 2 2<br />
= T3 = T1 + T2 T 1<br />
y<br />
T 2<br />
θθθθ<br />
T 3<br />
T 3 cosθθθθ<br />
T 3 sinθθθθ<br />
x
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Newton’s 2 nd Law:<br />
broken up into components:<br />
F Σ net = Fi = T1 + T2 + T3 = ma = 0<br />
i<br />
x: F Σ net,x = Fi,x = −T1 + T3 cosθθθθ = 0<br />
i<br />
� T 3 cosθθθθ = T 1<br />
y: F Σ net,y = Fi,y = −T2 + T3 sinθθθθ = 0<br />
i<br />
� T 3 sinθθθθ = T 2<br />
divide (2) by (1) to get:<br />
tanθθθθ = T 2 /T 1<br />
SMU PHYS1100, fall 2008, Prof. Clarke 6<br />
(1)<br />
(2)<br />
(1) 2 + (2) 2 �<br />
T3 cos2θθθθ + T3 sin2θθθθ = T3 (cos2θθθθ + sin2 2 2<br />
2<br />
θ θ θ θ )<br />
2 2 2<br />
= T3 = T1 + T2 T 1<br />
y<br />
T 2<br />
θθθθ<br />
T 3<br />
T 3 cosθθθθ<br />
T 3 sinθθθθ<br />
x
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Newton’s 2 nd Law:<br />
broken up into components:<br />
F Σ net = Fi = T1 + T2 + T3 = ma = 0<br />
i<br />
x: F Σ net,x = Fi,x = −T1 + T3 cosθθθθ = 0<br />
i<br />
� T 3 cosθθθθ = T 1<br />
y: F Σ net,y = Fi,y = −T2 + T3 sinθθθθ = 0<br />
i<br />
� T 3 sinθθθθ = T 2<br />
divide (2) by (1) to get:<br />
tanθθθθ = T 2 /T 1<br />
SMU PHYS1100, fall 2008, Prof. Clarke 7<br />
(1)<br />
(2)<br />
(1) 2 + (2) 2 �<br />
T3 cos2θθθθ + T3 sin2θθθθ = T3 (cos2θθθθ + sin2 2 2<br />
2<br />
θ θ θ θ )<br />
2 2 2<br />
= T3 = T1 + T2 T 1<br />
y<br />
T 2<br />
θθθθ<br />
T 3<br />
T 3 cosθθθθ<br />
T 3 sinθθθθ<br />
x
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Newton’s 2 nd Law:<br />
broken up into components:<br />
F Σ net = Fi = T1 + T2 + T3 = ma = 0<br />
i<br />
x: F Σ net,x = Fi,x = −T1 + T3 cosθθθθ = 0<br />
i<br />
� T 3 cosθθθθ = T 1<br />
y: F Σ net,y = Fi,y = −T2 + T3 sinθθθθ = 0<br />
i<br />
� T 3 sinθθθθ = T 2<br />
divide (2) by (1) to get:<br />
tanθθθθ = T 2 /T 1<br />
SMU PHYS1100, fall 2008, Prof. Clarke 8<br />
(1)<br />
(2)<br />
(1) 2 + (2) 2 �<br />
T3 cos2θθθθ + T3 sin2θθθθ = T3 (cos2θθθθ + sin2 2 2<br />
2<br />
θ θ θ θ )<br />
2 2 2<br />
= T3 = T1 + T2 T 1<br />
y<br />
T 2<br />
θθθθ<br />
T 3<br />
T 3 cosθθθθ<br />
T 3 sinθθθθ<br />
x
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Newton’s 2 nd Law:<br />
broken up into components:<br />
F Σ net = Fi = T1 + T2 + T3 = ma = 0<br />
i<br />
x: F Σ net,x = Fi,x = −T1 + T3 cosθθθθ = 0<br />
i<br />
� T 3 cosθθθθ = T 1<br />
y: F Σ net,y = Fi,y = −T2 + T3 sinθθθθ = 0<br />
i<br />
� T 3 sinθθθθ = T 2<br />
divide (2) by (1) to get:<br />
tanθθθθ = T 2 /T 1 = 1.2 � θθθθ = 50 o<br />
ended here, 25/9/08<br />
SMU PHYS1100, fall 2008, Prof. Clarke 9<br />
(1)<br />
(2)<br />
(1) 2 + (2) 2 �<br />
T3 cos2θθθθ + T3 sin2θθθθ = T3 (cos2θθθθ + sin2 2 2<br />
2<br />
θ θ θ θ )<br />
2 2 2<br />
= T3 = T1 + T2 = 24,400 � T3 = 156 N<br />
T 1<br />
y<br />
T 2<br />
θθθθ<br />
T 3<br />
T 3 cosθθθθ<br />
T 3 sinθθθθ<br />
x
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
If v = 0 (but constant since a = 0), equilibrium is dynamic.<br />
example: A car of weight 15,000 N is towed up a hill with an<br />
inc<strong>line</strong> of 20 o at constant velocity. Friction is negligible. The<br />
tow rope is rated at 6,000 N maximum tension. Will it break?<br />
Notice we have chosen the x-axis to point up the hill,<br />
and not horizontally. See rules of thumb on page 13.<br />
SMU PHYS1100, fall 2008, Prof. Clarke 10
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Newton’s 2 F Σ net = Fi i<br />
= T + n + w = ma = 0<br />
x: F Σ net,x = Fi,x = T −−−− w sinθθθθ = 0<br />
i<br />
nd Law:<br />
broken up into components:<br />
y<br />
� T = w sinθθθθ (1)<br />
y: F Σ net,y = Fi,y = n −−−− w cosθθθθ = 0<br />
i<br />
� n = w cosθθθθ (2)<br />
Since we are only asked about T, (2)<br />
is of no further interest to us. From<br />
(1), we have:<br />
T = (15,000) sin(20 o ) = 5,130 N<br />
Since T < 6,000 N, the rope does not break.<br />
SMU PHYS1100, fall 2008, Prof. Clarke 11<br />
w<br />
n<br />
θθθθ<br />
T<br />
w cosθθθθ<br />
θθθθ<br />
w sinθθθθ<br />
x
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Continuing with the same example, now suppose the tower<br />
grows impatient, and accelerates up the hill at 1.0 ms-2 .<br />
Whither the rope now?<br />
To the FBD, we add an acceleration<br />
vector. This changes the x-component<br />
of Newton’s 2 nd Law:<br />
x: F Σ net,x = Fi,x = T −−−− w sinθθθθ = ma<br />
i<br />
� T = w sinθθθθ + mg = w sinθθθθ + a a<br />
g ( g)<br />
� T = (15,000) sin(20 o ) + 1.0<br />
( ) 9.8<br />
= 6,660 N, and the rope breaks.<br />
SMU PHYS1100, fall 2008, Prof. Clarke 12<br />
y<br />
w<br />
n<br />
θθθθ<br />
T<br />
w cosθθθθ<br />
a<br />
θθθθ<br />
w sinθθθθ<br />
x
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Why did we choose an inc<strong>line</strong>d coordinate system in the<br />
previous example?<br />
Rule of thumb: Choose your coordinate system so that a lies<br />
<strong>along</strong> either the x-axis or the y-axis.<br />
- simplifies equations by having a appear in only one.<br />
Subordinate rule of thumb: If a = 0, choose your coordinate<br />
system so that most of the forces lie <strong>along</strong> either the x-axis or<br />
the y-axis.<br />
- reduces the task of resolving force vectors into x- and ycomponents.<br />
SMU PHYS1100, fall 2008, Prof. Clarke 13
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Clicker question 5.1<br />
Resolve T into its x- and ycomponents<br />
for the coordinate<br />
system shown.<br />
a) T x = T; T y = 0<br />
b) T x = 0; T y = T<br />
c) T x = T cosθθθθ; T y = T sinθθθθ<br />
d) T x = T sinθθθθ; T y = T cosθθθθ<br />
SMU PHYS1100, fall 2008, Prof. Clarke 14<br />
y<br />
θθθθ<br />
T<br />
x
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Clicker question 5.1<br />
Resolve T into its x- and ycomponents<br />
for the coordinate<br />
system shown.<br />
a) T x = T; T y = 0<br />
b) T x = 0; T y = T<br />
c) T x = T cosθθθθ; T y = T sinθθθθ<br />
d) T x = T sinθθθθ; T y = T cosθθθθ<br />
SMU PHYS1100, fall 2008, Prof. Clarke 15<br />
y<br />
θθθθ<br />
T x<br />
The x-component is adjacent<br />
to θθθθ and gets the cosθθθθ.<br />
The y-component is opposite<br />
to θθθθ and gets the sinθθθθ.<br />
T<br />
T y<br />
x
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Clicker question 5.2<br />
Resolve w into its x- and ycomponents<br />
for the coordinate<br />
system shown.<br />
a) w x = w; w y = 0<br />
b) w x = 0; w y = −w<br />
c) w x = −w cosθθθθ; w y = −w sinθθθθ<br />
d) w x = −w sinθθθθ; w y = −w cosθθθθ<br />
e) w x = w cosθθθθ; w y = −w sinθθθθ<br />
f) w x = w sinθθθθ; w y = −w cosθθθθ<br />
SMU PHYS1100, fall 2008, Prof. Clarke 16<br />
y<br />
θθθθ<br />
w<br />
x
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Clicker question 5.2<br />
Resolve w into its x- and ycomponents<br />
for the coordinate<br />
system shown.<br />
a) w x = w; w y = 0<br />
b) w x = 0; w y = −w<br />
c) w x = −w cosθθθθ; w y = −w sinθθθθ<br />
d) w x = −w sinθθθθ; w y = −w cosθθθθ<br />
e) w x = w cosθθθθ; w y = −w sinθθθθ<br />
f) w x = w sinθθθθ; w y = −w cosθθθθ<br />
Here, we had to see that θθθθ<br />
is also the angle between<br />
w and the y-axis, and that<br />
both components point in<br />
their negative directions.<br />
SMU PHYS1100, fall 2008, Prof. Clarke 17<br />
y<br />
θθθθ<br />
w<br />
θθθθ<br />
w y<br />
w x<br />
x
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Clicker question 5.3<br />
Resolve w into its x- and ycomponents<br />
for the coordinate<br />
system shown.<br />
a) w x = w; w y = 0<br />
b) w x = 0; w y = −w<br />
c) w x = −w cosθθθθ; w y = −w sinθθθθ<br />
d) w x = −w sinθθθθ; w y = −w cosθθθθ<br />
e) w x = w cosθθθθ; w y = −w sinθθθθ<br />
f) w x = w sinθθθθ; w y = −w cosθθθθ<br />
SMU PHYS1100, fall 2008, Prof. Clarke 18<br />
θθθθ<br />
w<br />
y<br />
x
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Clicker question 5.3<br />
Resolve w into its x- and ycomponents<br />
for the coordinate<br />
system shown.<br />
a) w x = w; w y = 0<br />
b) w x = 0; w y = −w<br />
c) w x = −w cosθθθθ; w y = −w sinθθθθ<br />
d) w x = −w sinθθθθ; w y = −w cosθθθθ<br />
e) w x = w cosθθθθ; w y = −w sinθθθθ<br />
f) w x = w sinθθθθ; w y = −w cosθθθθ<br />
SMU PHYS1100, fall 2008, Prof. Clarke 19<br />
θθθθ<br />
w y<br />
θθθθ<br />
w x<br />
It doesn’t matter whether we<br />
measure θθθθ between the x-axis<br />
and the horizontal, or<br />
between the y-axis and the<br />
vertical; it’s the same angle.<br />
Here, the x-component points<br />
in the +x direction.<br />
θθθθ<br />
w<br />
y<br />
x
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
A model for friction…<br />
Any two solid surfaces in contact will “stick” to each other, even if very<br />
weakly, via electrostatic bonds between molecules that come in very close<br />
proximity to each other. When the surfaces are at relative rest, these bonds<br />
have more time to set up than when the<br />
surfaces are in relative motion.<br />
Thus, the maximum static friction, f s,max ,<br />
ought to be greater than the kinetic<br />
friction, f k .<br />
SMU PHYS1100, fall 2008, Prof. Clarke (figures from Doug Davis @ Eastern Illinois University) 20
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Even though the detailed physics of two surfaces in contact is very<br />
complex, experiments reveal a very simple model for friction.<br />
Consider a block at rest on the surface of a table. Gradually increase the<br />
horizontal force, F pull , on the cord. When F pull reaches a critical level, f s,max ,<br />
the block suddenly moves. The frictional force, f, reduces to f k and remains<br />
constant, regardless of how much higher F pull gets.<br />
Experimentally, one finds f s,max = µµµµ s n and f k = µµµµ k n where µµµµ s > µµµµ k are the<br />
coefficients of static and kinetic friction respectively (Table 5.1, pg. 133).<br />
n<br />
F pull<br />
F pull<br />
SMU PHYS1100, fall 2008, Prof. Clarke 21<br />
f<br />
f s,max<br />
f k<br />
0 static region kinetic region
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Note that f s is not always µµµµ s n. When F pull = 0, f s = 0 too.<br />
f s,max= µµµµ sn is the maximum static<br />
frictional force the surface can exert<br />
before the “sticking” molecular bonds<br />
break, and after which the kinetic<br />
frictional force takes over.<br />
The direction of f is tangential to the<br />
surface, and thus perpendicular to n.<br />
The direction of f s is opposite to the<br />
motion it is preventing.<br />
The direction of f k is opposite to the<br />
velocity.<br />
velocity frog would have if<br />
there were no f s<br />
SMU PHYS1100, fall 2008, Prof. Clarke 22
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
example for the board (e.g. 5.6 from text): A 50 kg box is<br />
pushed across the floor (µµµµ k = 0.20) at a steady speed of 2.0 ms -1 .<br />
a) How much force is exerted on the box?<br />
b) If the force is stopped, how far will the box slide <strong>along</strong> the<br />
floor before coming to rest?<br />
notes 5.1<br />
SMU PHYS1100, fall 2008, Prof. Clarke 23
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
example for the board (e.g. 5.7 from text): A 50.0 kg steel filing<br />
cabinet is in the back of a dump truck. The steel bed of the<br />
truck (µµµµ s = 0.80) is slowly raised.<br />
a) What is the magnitude and direction of the static frictional<br />
force when the bed is tilted by 20 o ?<br />
b) At what angle does the cabinet begin to slide?<br />
notes 5.2<br />
SMU PHYS1100, fall 2008, Prof. Clarke 24
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Clicker question 5.4<br />
Consider the static or kinetic frictional force, if any, between the box and<br />
table when the table is tilted as shown in the four cases. The box is<br />
stationary in cases 1, 2, and 3 with the box just on the verge of slipping in<br />
case 3. The box slides with constant speed in case 4.<br />
Which of the following is true?<br />
a) 0 < f s,1 < f s,2 < f k,4 < f s,3 = µµµµ s n b) 0 = f s,1 < f s,2 < f k,4 < f s,3 = µµµµ s n<br />
c) µµµµ k n = f k,4 < f s,1 = f s,2 = f s,3 = µµµµ s n d) 0 = f s,1 < f k,4 < f s,2 = f s,3 = µµµµ s n<br />
f s,1<br />
f s,2<br />
1 2<br />
3<br />
4<br />
SMU PHYS1100, fall 2008, Prof. Clarke 25<br />
f s,3<br />
f k,4<br />
v
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Clicker question 5.4<br />
Consider the static or kinetic frictional force, if any, between the box and<br />
table when the table is tilted as shown in the four cases. The box is<br />
stationary in cases 1, 2, and 3 with the box just on the verge of slipping in<br />
case 3. The box slides with constant speed in case 4.<br />
Which of the following is true?<br />
a) 0 < f s,1 < f s,2 < f k,4 < f s,3 = µµµµ s n b) 0 = f s,1 < f s,2 < f k,4 < f s,3 = µµµµ s n<br />
c) µµµµ k n = f k,4 < f s,1 = f s,2 = f s,3 = µµµµ s n d) 0 = f s,1 < f k,4 < f s,2 = f s,3 = µµµµ s n<br />
f s,1<br />
f s,2<br />
1 2<br />
3<br />
4<br />
SMU PHYS1100, fall 2008, Prof. Clarke 26<br />
f s,3<br />
f k,4<br />
v
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Clicker question 5.5<br />
You push lightly against the computer sitting on your desk<br />
and it doesn’t move. You push harder still, and it still won’t<br />
budge. This is because…<br />
a) The friction between the computer and the table<br />
increases the harder you push.<br />
b) The amount of friction is constant, but you haven’t<br />
pushed hard enough to overcome this force.<br />
c) The friction between the computer and the table<br />
decreases the more you push.<br />
d) None of the above.<br />
SMU PHYS1100, fall 2008, Prof. Clarke 27
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Clicker question 5.5<br />
You push lightly against the computer sitting on your desk<br />
and it doesn’t move. You push harder still, and it still won’t<br />
budge. This is because…<br />
a) The friction between the computer and the table<br />
increases the harder you push.<br />
b) The amount of friction is constant, but you haven’t<br />
pushed hard enough to overcome this force.<br />
c) The friction between the computer and the table<br />
decreases the more you push.<br />
d) None of the above.<br />
SMU PHYS1100, fall 2008, Prof. Clarke 28
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Mass, weight, and apparent weight<br />
mass, m, is a measure of the quantity of matter (kg).<br />
weight, w, is the gravitational force exerted on m (N). w = mg<br />
Take the earth away and your weight, w, disappears, but the amount of<br />
matter in your body, m, remains the same.<br />
apparent weight, w app, is the magnitude of the contact force<br />
that supports the weight, w.<br />
e.g., when you stand on a scale, the scale actually measures the normal<br />
force preventing you from falling through the scale. The normal force that<br />
registers on the dial is your apparent weight.<br />
Often, w app = w. However, if m is accelerating in a direction parallel to g,<br />
w app will not be the same as w.<br />
SMU PHYS1100, fall 2008, Prof. Clarke 29
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
e.g., a man stands on a (spring) scale in an elevator<br />
accelerating upward with acceleration a. Find his<br />
apparent weight, F sp.<br />
F Σ net = Fi,y = Fsp − w = ma<br />
i<br />
a<br />
� Fsp = w + ma = w + mg g<br />
� Fsp = w 1 + a ( g)<br />
exercise: show that if the elevator is<br />
accelerating downward instead, the<br />
man’s apparent weight is given by:<br />
Fsp = w ( 1 −−−−<br />
a) g<br />
ended here, 30/9/08<br />
SMU PHYS1100, fall 2008, Prof. Clarke 30
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Weightlessness is when the apparent weight is zero, not the<br />
weight itself.<br />
e.g., what is the weight and apparent weight<br />
of a rock in free-fall?<br />
w = mg = 0 is the only force acting on m,<br />
(ignoring drag) and causes the rock to<br />
accelerate downward with acceleration g.<br />
Thus:<br />
wapp = w (<br />
g<br />
1 −−−− g)<br />
= 0<br />
So, when m is in freefall, w app = 0 and we say m is weightless<br />
even though its actual weight is not zero. The term weightless<br />
is evidently a bit of a misnomer.<br />
SMU PHYS1100, fall 2008, Prof. Clarke 31<br />
m<br />
mg<br />
g
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Air drag. Like friction, air drag is a<br />
very complicated process. However,<br />
experiments show that a relatively<br />
simple expression for the air drag<br />
force, D, usually suffices:<br />
D = bAv 2<br />
where b is the drag coefficient = 0.25<br />
kg s -1 for air, A is the “presentation<br />
cross section area” of the object, and v<br />
is its speed relative to the air.<br />
The concept of “presentation cross<br />
section area” is illustrated to the right.<br />
SMU PHYS1100, fall 2008, Prof. Clarke 32
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Terminal speed. Because air drag increases with velocity<br />
(in fact, as v 2 ), a falling object will reach a speed, v term , such that<br />
D = mg. Here, the net force on m is zero,<br />
a = 0, and m falls at the constant and socalled<br />
terminal speed, v term, given by:<br />
D = bAv 2<br />
term= mg � vterm= with b set to ¼ for air.<br />
4mg<br />
A<br />
e.g., for a skydiver of mass m = 75 kg<br />
“laying flat”, A = 1.8 m x 0.4 m = 0.72 m 2 ,<br />
� v term = 64 ms −1 (230 km/hr)<br />
e.g., skydiver “standing”, A = 0.3 m x 0.4 m<br />
w = mg<br />
= 0.12 m<br />
SMU PHYS1100, fall 2008, Prof. Clarke 33<br />
2 � vterm = 157 ms−1 (560 km/hr)<br />
m<br />
D<br />
F net = 0<br />
v term
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
example for the board (Fig. 5.21<br />
from text)<br />
A ball of mass m and radius r<br />
moves up and down vertically.<br />
In the absence of air drag, the<br />
ball would be in free-fall with<br />
acceleration –g.<br />
Including air drag,<br />
a) what is the acceleration of<br />
the ball on the way up?<br />
b) what is the acceleration of<br />
the ball on the way down?<br />
notes 5.3<br />
SMU PHYS1100, fall 2008, Prof. Clarke 34
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
example for the board (e.g.,<br />
5.10 from text)<br />
A wooden sled with mass (including<br />
rider and supplies) 200 kg is pulled<br />
by a dog team over snow (µµµµ k = 0.06)<br />
with two ropes (inc<strong>line</strong>d at θθθθ = 10 o to<br />
the horizontal) attaching the sled to<br />
the team. It takes the dogs 15 metres<br />
to reach their cruising speed of 5 ms -1 .<br />
a) What is the tension in the ropes while the dogs<br />
are accelerating (assumed to be constant)?<br />
b) What is the tension in the ropes at cruising<br />
speed?<br />
notes 5.4<br />
SMU PHYS1100, fall 2008, Prof. Clarke 35
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
example for the board (e.g., 5.11 from text)<br />
A 100 kg box rests on the floor of a flatbed truck. The coefficients of<br />
friction between the box and the floor are µµµµ s = 0.3 and µµµµ k = 0.2.<br />
a) What is the maximum acceleration the truck can have without causing<br />
the box to slip?<br />
notes 5.5<br />
b) Suppose the truck is accelerating at this maximum acceleration and a<br />
bump causes the box to start slipping. If the distance between the centre<br />
of the box and the end of the flatbed is 3.0 m, how long does it take for<br />
the box to fall off the end of the truck?<br />
SMU PHYS1100, fall 2008, Prof. Clarke 36
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Clicker question 5.6<br />
An elevator is going UP at constant speed. The only two forces<br />
on the elevator are tension, T, from a cable pulling UP, and the<br />
weight of the elevator and its contents, w, pulling DOWN. How<br />
does the magnitude of T compare to the magnitude of w?<br />
a) T > w<br />
b) T = w<br />
c) T < w<br />
d) not enough information to tell<br />
SMU PHYS1100, fall 2008, Prof. Clarke 37
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Clicker question 5.6<br />
An elevator is going UP at constant speed. The only two forces<br />
on the elevator are tension, T, from a cable pulling UP, and the<br />
weight of the elevator and its contents, w, pulling DOWN. How<br />
does the magnitude of T compare to the magnitude of w?<br />
a) T > w<br />
b) T = w<br />
c) T < w<br />
d) not enough information to tell<br />
SMU PHYS1100, fall 2008, Prof. Clarke 38
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Clicker question 5.7<br />
Consider the tension in the cable holding up an elevator<br />
under the following three situations:<br />
1) the elevator is stationary;<br />
2) the elevator is being accelerated upwards at 2.0 ms −2 ;<br />
3) the elevator is being accelerated downward at 2.0 ms −2 ;<br />
Rank these situations from greatest tension to lowest tension<br />
in the cable:<br />
a) 2,1,3 b) 2,3,1 c) 3,1,2 d) all the same<br />
SMU PHYS1100, fall 2008, Prof. Clarke 39
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Clicker question 5.7<br />
Consider the tension in the cable holding up an elevator<br />
under the following three situations:<br />
1) the elevator is stationary;<br />
2) the elevator is being accelerated upwards at 2.0 ms −2 ;<br />
3) the elevator is being accelerated downward at 2.0 ms −2 ;<br />
Rank these situations from greatest tension to lowest tension<br />
in the cable:<br />
a) 2,1,3 b) 2,3,1 c) 3,1,2 d) all the same<br />
SMU PHYS1100, fall 2008, Prof. Clarke 40
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Clicker question 5.8<br />
Now consider the tension in the cable holding up an elevator<br />
under the following three situations:<br />
1) the elevator is stationary;<br />
2) the elevator is moving upwards at constant speed;<br />
3) the elevator is moving downward at constant speed;<br />
Rank these situations from greatest tension to lowest tension<br />
in the cable:<br />
a) 2,1,3 b) 2,3,1 c) 3,1,2 d) all the same<br />
SMU PHYS1100, fall 2008, Prof. Clarke 41
<strong>Chapter</strong> 5: <strong>Motion</strong> <strong>along</strong> a <strong>line</strong><br />
Clicker question 5.8<br />
Now consider the tension in the cable holding up an elevator<br />
under the following three situations:<br />
1) the elevator is stationary;<br />
2) the elevator is moving upwards at constant speed;<br />
3) the elevator is moving downward at constant speed;<br />
Rank these situations from greatest tension to lowest tension<br />
in the cable:<br />
a) 2,1,3 b) 2,3,1 c) 3,1,2 d) all the same<br />
SMU PHYS1100, fall 2008, Prof. Clarke 42